Interference and DIffraction

Introduction

The wave-particle duality in light states that matter and light exhibits the behaviors of both waves and particles, depending upon the circumstances of the experiment. Certain physical phenomena are most easily explained by invoking the wave nature of light, rather than the particle nature.  Thomas Young's double slit experiment resulted in obvious wave behavior and seemed to firmly support the wave theory of light. Interference phenomena prove the wave nature of light. Interference refers to superposition of two or more waves that meet at one point in space. Waves encountering each other arise to two possibilities of formation. A condition wherein the waves combine and add up corresponds to constructive interference whereas the waves cancelling out each other correspond to destructive interference. Constructive interference occurs when two waves are in phase. To be in phase, the points on the wave must have Δφ=(2 π)m, where m is an integer. Destructive interference occurs when two waves are a half cycle out of phase. To be out of phase the points on the wave must have Δφ=(2 π)(m+½), where m is an integer. Diffraction is used to describe the interference pattern that results from a slit with no negligible width. Huygens’s principle states that every point on a wave front is a source of wavelets; light will spread out when it passes through a narrow slit. Diffraction is applicable only when the slit width is nearly the same size or smaller than the wavelength.
            Light bends around obstacles like waves do, and it is this bending that causes the single-slit diffraction pattern. The pattern is composed of small slits, relative to the wavelength of light, with alternating dark and bright fringes visible from a screen placed far away. Most of the light is concentrated in the broad central diffraction maximum. The minor secondary bands are located at either sides of the central maximum. The first diffraction minimum occurs at the angles given the equation: sin Ɵ = ƛ / a where a is the width size. Waves passing through one of two narrow slits will diffract in passing through each slit and will result to an interference with the waves from the other slit resulting to a double-slit interference.

The objectives of this experiment are to be able to investigate the patterns produced by diffraction through a single slit, to quantitatively relate the single-slit diffraction pattern obtained to the slit width, to differentiate the patterns produced by single-slit diffraction and double-slit diffraction, to quantitatively relate the double-slit diffraction pattern to the slit width, and to determine the qualitative relationship between a double-slit diffraction pattern and the corresponding slit separation.

Methodology

The experiment was setup with a laser placed at one end of an optics bench together with a single slit disk 3 cm in front of the laser. A white sheet of paper which the light should be projected on was also attached in front of the optics bench. The room lights were turned off and the only sources of light allowed was from the laser diode and a desk lamp. The first part of the experiment was the observation of a single slit diffraction wherein a 0.04 mm width single slit was used. The laser was then turned on and lines of red light with several dark fringes were projected on the white paper. The distance between the first-order minima as well as that of the second-order minima were measured using  a rule. The obtained distances were  then halved in order to calculate the distances of each  minima from the center. The same procedure was repeated after changing the slit width from 0.04 mm to 0.02 mm. The slit width was changed to 0.08 mm but only for the observation and sketching of its diffraction pattern. Using the obtained measurements, the experimental wavelength was then calculated.

The next part of the experiment was the observation of a double-slit interference wherein the setup was still the same as that from the first part but this time, a double slit was used. A double-slit with a 0.04 mm slit width and a slit separation varying from 0.125 mm to 0.75 mm was used after which, a qualitative observation was done on its interference fringes as well as its diffraction envelope as the slit separation was varied. The slit separation was then changed to 0.25 mm. The locations of the intensity minima that was projected on the paper were marked and the distances between the first-order minima and that of the second-order minima were recorded which were then halved in order to obtain their distances from the center. 

 Using the same double-slit width and slit separation, the interference pattern was again projected on the white paper. The width of the central maximum was measured and the number of interference fringes located inside it was also counted, with the data used to calculate the approximate value of the width of each interference fringe. The same procedure was repeated but this time, the following double-slits were used:

●        a=0.04 mm, d=0.50 mm

●        a=0.08 mm, d=0.25 mm

●        a=0.08 mm, d=0.50 mm

Set-up of the Experiment

Materials

 Results and Discussion

A.    Single-slit Diffraction

In this part of the experiment, the laser, the light source for the entire experiment, was made to pass through a single slit and project at a white surface at a fixed distance of 0.9 meters from the slit, which is the slit-to-screen distance given by L. Upon projection of the light, first passing through a single slit of slit width 0.02mm, given by a, and having the value of m equal to one, the pattern found on the surface was a series of bright and dark fringes. The bright fringes, maxima, and the dark fringes, minima, appeared fainter from the center of the projected pattern. This diffraction pattern for the single-slit is called the diffraction envelope. 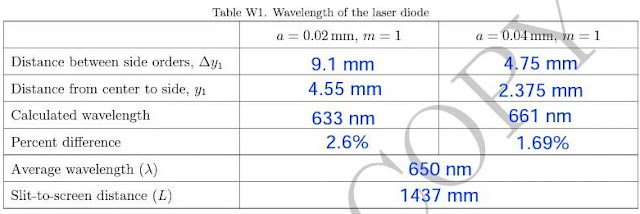After the diffraction envelope was traced, the distance between the side orders, Δy_1, which is the distance between the center of the first dark-fringe to the left of the central maximum and the first dark-fringe to the right of the central maximum, was measured using a ruler. The distance from the center to the side, y₁, was also measured by dividing Δy₁, by two. The wavelength was calculated using the formula for locating the m^th intensity minimum from the center of the pattern:

                                                                       

where y_m,diff in this case is y_1, and lamda is the wavelength of the laser light source.¹ Solving for the wavelength, the formula: 

was used. The values for Δy_1, y_1, and λ were also obtained for a larger slit width of 0.04mm. The results obtained are shown in the Table W1.

The wavelength of the laser light source is theoretically 6.50x10-⁹m. The obtained wavelength from the data table above shows that there is a 1.7 - 2.6 percent difference from the theoretical value of the wavelength that is negligible. This may be due to certain sources of error which will be discussed in the latter part of the discussion, however the measurement of the wavelength in this part of the experiment should have been consistent as the light source remained constant. The data above showed that the distance between the side orders and consequently, the distance from center to side, decreased as the slit width became larger. Consistent with the formula, the slit width in a single-slit diffraction is inversely proportional to the distance between side orders and the distance from the center to side.

Figure 1, Single-slit diffraction

A.    Double-slit Interference

In double-slit interference, a diffraction envelope is also observed combined with a series of alternating bright and dark fringes inside the diffraction envelope. The distance between the side orders, the distance from the center to the side, and the wavelength was measured with a fixed slit-to-screen distance of 1437 mm and a fixed slit width of 0.04 mm, and slit separation of 0.25 mm for the double-slit. The projected light was traced and the distance between side orders and the distance from the center to the side was measured using a ruler. The formula

which is similar in form with the previous equation, with the slit separation d. However, they are different in principle , the previous equation gives the position of intensity minima in a single slit setup, while that equation gives the position of intensity maxima in a double-slit setup.

Also, the equation also gives the width of each fringes, bright or dark.

Solving for the d or the distance between the two narrow slit

where lamda is the wavelength, L is the slit-screen distance and ym is the width of the formed fringe. The results are recorded in the TW3.

        Similar to the data from the single-slit diffraction, the distance between side orders increased proportionally as m was increased. The distance between the side orders and distance from the center to the side for a double-slit diffraction pattern is directly proportional, and the data obtained is seen to be consistent. The obtained d had a percent difference of 3.2-11.2 as compared to the theoretical, and this may have also been due to certain sources of error.

After which, the values for the slit width and the slit separation were varied and the number of fringes inside the central maximum and the width of the central maximum was measured in order to calculate for the fringe width of each fringe inside the central maximum, in which all are equal in length. The fringe width was calculated by dividing the width of the central maximum by the number of fridges. The results are shown in the data table below. 

The table W4 illustrates the trend that the number of fringes increases as the slit separation is increased. It can also be seen that the number of fringes decreases as the slit width is increased. From this, it can be said that the number of fringes is directly proportional to the slit separation and is inversely proportional to the slit width. 

Also the table W4 shows that the width of the central maximum didn’t change or exhibited negligible change when the slit separation was varied with the slit width kept constant. However, the width of the central maximum decreased when the slit width was doubled. From this, it can be said that the width of the central maximum is not affected by the slit separation and is inversely proportional to the slit width.

The calculated fringe width is seen to decrease as the slit width is increased, regardless if the slit separation is 0.25mm or 0.50mm. The calculated fringe width also decreases as the slit separation is increased. I can conclude that the fringe width is inversely proportional to both the slit width and the slit separation. 

Figure 2. Double-slit Interference (a=0.04 mm, d=0.25 mm)

Figure 3. Double-slit Interference (a=0.04 mm, d=0.50 mm)

Figure 4. Double-slit Interference (a=0.08 mm, d=0.25 mm)

Figure 4. Double-slit Interference (a=0.08 mm, d=0.50 mm)

Conclusions

Based on our data and results, the pattern produced by single-slit diffraction, called diffraction envelope, was an alternating band of bright and dark fringes, which is symmetrical over the center of the projected pattern. In the single-slit diffraction pattern, an increase in the slit width, while keeping the slit-to-screen distance and light source constant, contributed to a decrease in the distance between side orders. An increase in the value of m contributed to a proportional increase to the distance between side orders. In double-slit diffraction, the pattern was a diffraction envelope with a series of bright and dark fringes in the diffraction envelope. An increase in the value of m in double-slit diffraction contributed to a proportional increase to the distance between side orders. An increase in the value of the slit separation, while keeping the slit width constant, contributed to an increase in the number of fringes in the diffraction envelope, and an increase in the slit width contributed to a decrease in the number of fringes and width of the central maximum. Despite having attained significant percent errors for the values of the wavelength, which may have been contributed by the source of error, the results of the group observed the patterns and trends of the theoretical single-slit and double-slit diffraction. For more accurate results, it is recommended that the experiment be performed in a room well isolated from external light sources which could affect the experimental results.

References

1.       “Experiment 8 Interference and Diffraction,” Laboratory Manual for Physics (Physics 72.1), (2013).

2.       C. Colwell, “Physical Optics - Interference and Diffraction Patterns,” taken from: http://dev.physicslab.org/document.aspx?doctype=3&filename=physicaloptics_interferencediffraction.xml

3.       D. Morin, “Chapter 9 Interference and Diffraction,” Waves (to be published)