Gas Laws

The behavior of gas under different pressures, volumes and temperatures have been widely studied in the history of Physics. In 1662, Robert Boyle discovered that close to room temperature and at about one atmospheric pressure, the pressure and volume of gases are inversely proportional to each other and this relationship became known as Boyle’s Law. Boyle's Law is written as

where the proportionality constant CB is NkT.

Jacques Charles discovered in 1780 that close to room temperature and at about one atmospheric pressure, the volume and temperature of gases are directly proportional to each other. Known today as Charles’s Law, which is written as 

where the proportionality constant CC is Nk/P.

The Gay-Lussac’s Law states that  close to room temperature and at about one atmospheric pressure, the pressure and temperature of gases are directly proportional to each other. It was discovered by Joseph Louis Gay-Lussac in 1809 and named after him. Gay-Lussac’s Law is written as 

where the proportionality constant CG = Nk/V.

These laws can be explained if we assume that the gas is ideal. The ideal gas model is used to describe the behavior of dilute gases at low pressures and high temperatures. Many gases e.g., nitrogen, oxygen and hydrogen, can be considered to be ideal gases at room temperature and pressures close to 1 atm. Using this assumption, combining the three gas laws discuss above, which is discovered experimentally gives as the equation of the state of an ideal gas.

where P is the pressure, V is the volume, N is the number of particles and T is the temperature of the gas measured in the absolute or Kelvin scale. The constant k in the ideal gas equation is called the Boltzmann constant and has the value k = 1.3806488×10−23 m^2·kg/s^2·K^1.

In this experiment, we wish to verify the Boyle's Law and Charles's Law only and  use the ideal gas equation to compute for the number of particles in the gas.

Boyle's Law

First, a beaker was filled with water up to 3/4 full and the water was boiled. The water was boiled throughout the activity. The air chamber can was then connected to the mass lifter apparatus using the rubber tubing. The air chamber can was then placed in the hot bath. The piston was lifted to its maximum height and the gas pressure sensor was connected to the Vernier LabQuest. The temperature T was monitored throughout the experiment. A 50 g standard mass was placed on the platform of the mass lifter apparatus and the height h of the piston and the pressure P was recorded. The previous step was also done for the 100,150,200 and 250 g masses.

The height of the gas in the piston where recorded. The volume of the cylinder Vcyl for each height was computed. The reciprocal P of each pressure reading was computed. Plotting  Vcyl vs P^-1 was done. 

Table B1

Table 1 above shows us that as we increase the mass of the object that we place, the height decreases and the pressure increases as well.

Figure 1

The information that we got from the linear fit equation are the following:

We obtain the volume of the chamber which is .0004 m^3, and also we can get the number of particle using the slope of the linear fit which is 1.0543E+22.

Charles's Law

The first step in this part of the experiment was to remove the beaker  (will serve as the hot bath) from the top of the stove. The air chamber was placed in the hot bath. The pressure P was monitored throughout the experiment. The temperature T of the hot bath was measured using a digital thermometer and the initial height h of the piston was recorded. 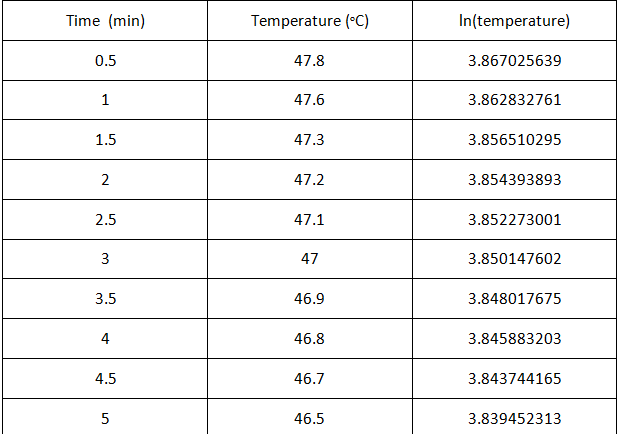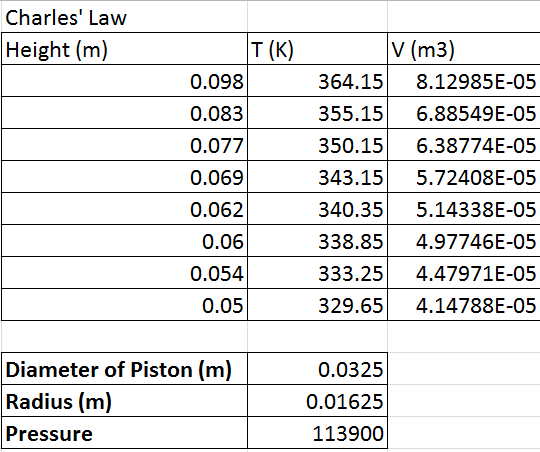Chunks of ice were slowly added on the hot bath while taking h and T measurements at equal time intervals. A graph of Vcham VS Temperature using the obtained data below. Also, the data from table 2 tells us that as the temperature decreased, the height decreased which means that the volume decreased.

Table 2A

Figure 2

Table 2B

 The number of particles N, which is 8.2487E+21 and the volume of the air chamber can Vcham is .0003 m^3 were calculated from the slope and y-intercept, respectively.

Conclusion: The data is precise because we have R^2 = .99 on both experiment. The data on the two experiment has only small discrepancy, since the theoretical number of particles is not given, the data obtained cannot be verified if its accurate. Over all, the experiment is well done with a small discrepancy.

Reference:

1.       “Experiment 9 Gas Laws,” Laboratory Manual for Physics (Physics 73.1), (2013)

Calorimetry

The objective of this experiment is to determine the specific heat of different metal samples aluminum and copper. The amount of heat required to change the temperature of an object depends on its mass m, the change in temperature ΔT, and the specific heat capacity c. 

The specific heat capacity is an intrinsic property of an object, and depends on the material that the object is made of. For water, the specific heat capacity is 4.184 J/(g C°).

To measure the specific heat of an object, we can first heat it to some known temperature, say the boiling point of water. Then, we transfer the object to a water bath of known mass and initial temperature. Finally, we measure the final equilibrium temperature of the system (the object, the water in the bath, and the water-bath container). If the system is thermally isolated from its surroundings (by insulating the container, for example), then the heat released by the object will equal the heat absorbed by the water and the container. This process is called calorimetry, and the insulated water container is called calorimeter.

A calorimeter is composed of an insulated vessel, which prevents heat released by a thermal interaction inside the vessel to escape into the surroundings. In this activity, the calorimeter consists of stacked coffee cups. The calorimeter is partially filled with tap water. The sample is then mixed into the tap water and the ΔT of the tap water is measured. If the calorimeter initially has the same temperature as the tap water, the amount of heat lost by the hot water is equal to heat absorbed by the tap water and the calorimeter: 

The experiment is split into two parts. In the first part, the heat capacity of the calorimeter will be determined.

 This information will then be used in the second part, the determination of the specific heat capacity of metal samples. 

HOT: 82 oC – 83g
TAP: 29.1 oC – 68g
Plugging in the values,
Trial 1, Tf = 56.09  => qgained-calorimeter 1318.84 J => Ccal = 48.86 J/ oC

HOT: 81 oC – 54g
TAP: 29.1 oC – 84.7g
Trial 2, Tf = 47.8,   q = 874.07  =>  Ccal = 46.74 J/ oC

HOT: 71.2 oC – 70.9g
TAP: 29.4 oC – 75.4g
Trial 3,  Tf = 48.96  q = 426.73 C =  21.82 J/ oC (dont know bakit siya lumayo maybe last trial, and naalala ko minadali na namin to kasi kukunin na yung hotplate :( )

So we get 48.86 J/ oC, 46.74 J/ oC, and 21.89 J/ oC values for Ccal. So the Ccal average is 39.14 J/ oC. Since the theoretical value is not given we don't know if our data is accurate (malamang malayo kasi di precise e).

Next part of the experiment is the calculation of the specific heat of two given metal, aluminum and copper using the computed Ccal. Unfortunately our data here are nowhere to be found (seryoso po mam) huhuhu. (may mga bagay talagang hindi nagpapaalam na mawawala kusa na lang itong maglalaho huhuhu ). The calculation for the specific heat of aluminum and copper are the same as with Ccal. Plug and play lang, given ka na ng lahat specific heat of aluminum at copper na lang yung unknown. From the experiment you can get the Final temperature and initial temperature reading and the masses. Tapos ayon plug and play na lang.

Reference
1.       “Experiment 7 Temperature Measurement,” Laboratory Manual for Physics (Physics 73.1), (2013).
2.      Tipler, P., Mosca, G. Physics for Scientists and Engineers. Chapter 25. W. H. Freeman and Company, New York, 2008.

Temperature Measurement

  One of the interesting principal branches in physics is thermodynamics, which is study of energy transformations involving heat, mechanical work, and other aspects of energy and how these transformations relate to physical properties. Thermodynamics can study microscopically that talks about the behavior of individual atoms and molecules and the other one is macroscopically properties in bulk such as volume, pressure, and etc..

      Temperature is one of the central concepts of thermodynamics. It gauges the "hardness" and "coldness" of a system. Thermometer is the device use to measure the temperature. It works by the principle of thermal equilibrium, which means further interaction between the body & the thermometer causes no further changes. In layman's term when two objects, one warm and one cold, are placed in contact with each other, the warmer object cools while the cooler object warms up. Eventually, no more changes in the warmness or coldness would occur and they would have the same temperature.

Zeroth Law of Thermodynamics
http://www.meritsection.com/class11/physics/thermodynamics/

      The concepts of thermal equilibrium was formally explained by the zeroth law of thermodynamics. Consider three systems A, B, and C. Also, a conductor permits thermal interactions between systems and an insulator prevents the thermal interactions between the two systems (this is an ideal insulator, in real world, an insulator also permits thermal interactions slowly). In the first picture, A&B is separated by an insulator(ideal), A&C and B&C are separated by conductor. Since, conductor permits the thermal interactions, in a time t A&C and B&C will be at thermal equilibrium. Therefore, A and B are also in thermal equilibrium with each other. In the second picture says that A&B will be at thermal equilibrium with each other at time t, and both A and B will not be in thermal equilibrium with C because they don't have any thermal interactions.

         Heat from the hotter object will transfer to the colder one until they both reach a common temperature that is different from their original temperatures. If a thermometer is placed in thermal contact with a hot body, what we actually read is the temperature of the thermometer itself! The act of touching the body with a thermometer changes the temperature of the body. It is therefore necessary that the transfer of heat to or from the thermal sensor is minimal such that it does not change the temperature of the object significantly. We have to wait until thermal equilibrium is reached before we can reliably read the temperature and it takes time to achieve this. How fast a thermal sensor achieves thermal equilibrium depends on the thermal time constant τ of the sensor.
          Consider a temperature sensor that has a reading T(t) at any time t. Initially, the sensor has a temperature T(t = 0) = Ti and is placed in contact with an object that is maintained at a constant temperature. After a sufficient time, the temperature sensor would have a final reading Tf. The difference between Tf and T(t) is ∆T(t) = Tf −T(t). As time progresses, the difference between the sensor reading and its final reading vanishes. If the sensor is a first-order linear device, the rate of change of ∆T can be assumed to be proportional to the difference of Ti and Tf,

d∆T dt = −k∆T. (1)

where k is a positive constant. The negative sign here implies that the rate of change of ∆t decreases in time. The left side of Equation (1) has dimensions [Temperature]/[Time]. To keep the dimensions the same as on the right side, the constant k must have dimensions of 1/[Time]. If we let k = 1/τ, where τ has units of time. Equation (1) becomes

d∆T dt= −∆T τ. (2)

Solving for T(t) we obtain,

T(t) = Ti + (Tf −Ti)(1−e^(−t/τ)).

        Temperature can be felt but cannot be measured directly. Instead we measure the degree of change in the properties of a material to heat and cold. For example, metals expand when heated. We can then measure how much the expansion is and equate it to a certain temperature. This and other measurable responses to heat are called thermometric properties.

        In this experiment we will using different temperature sensors and we will be interested with its thermal time constant, and this is dependent to the thermometric properties of the material.

       The experiment is divided in two procedure, the heating procedure and the cooling procedure. Basically, the two procedure is the same but will have different initial and final temperature.  First, we need a beaker or a pot with water up to 3/4 full and let the water boil. Keep the water boiling throughout the activity.  Take the thermometer and dip it in hot water. When the temperature stops increasing, record the final temperature as Tf in the worksheet. Take the thermometer and dip it in ice water. Wait for the temperature reading to stop decreasing then record it as Ti in the worksheet.  Compute the values T(τ) and tabulate in the worksheet. With initial temperature Ti, dip the thermometer in the boiling water then measure the time it takes for the reading to reach each T(τ). Record these values in the worksheet. Repeat the process three times each of the temperature sensor.

       The table above shows the data recorded from the experiment with three trials. The table above tells that the data is precise, the heating time constant recorded is 5.8 for heating and 6.0 for cooling in alcohol thermometer. Using alcohol thermometer, it takes six seconds before it reach thermal equilibrium. In simple words, approximately six seconds contact time is needed before we read the alcohol thermometer reading to be sure that its already the right temperature of the object we are measuring.

       The second table is the recorded data with three trials. Compare to the previous thermometer which is alcohol thermometer, mercury thermometer has approximately two seconds before it will reach thermal equilibrium. Therefore, mercury thermometer will have faster results compare to the alcohol thermometer. If we want to have a faster reading of the temperature we are testing it is advisable to use mercury thermometer than alcohol thermometer.

        The third table shows the data with three trials for thermocouple. The data shows that thermocouple only required approximately .2 seconds before it reach thermal equilibrium. If we want to measure a temperature with a fluctuating temperature it is better to use thermocouple because as fast as .2 second it will give you the reading of the object your trying to measure. Thermocouple has the least time constant because its operate with electric current which is very fast.

         The last table shows the time constant of each temperature measurement devices. The table shows that the thermocouple has least time constant which is .2 seconds, followed by the mercury thermometer with approximate 2 seconds and the last is alcohol thermometer with time constant approximately 6 seconds.

P.S. Hindi ko magraph yung exponential function using its equation, hindi ako makahanap ng app
kaya walang graph huhuhu

Reference
1.       “Experiment 7 Temperature Measurement,” Laboratory Manual for Physics (Physics 73.1), (2013).
2.       Walker, J., Halliday, D., & Resnick, R. (2011). Fundamentals of physics. Hoboken, NJ: Wiley.